Software Lab 6.3 Solutions

Iain Pardoe

Lesson 6.3: Multiple Linear Regression

Software Lab 6.3 Solutions

Males tend to receive slightly higher evaluation scores, although there is a lot of overlap. Scores for males range from 2.67 to 4.88 with a mean of 4.17 and a standard deviation of 0.423. Scores for females range from 2.30 to 4.74 with a mean of 3.96 and a standard deviation of 0.523.

Figure 1: Boxplots showing score by gender: (left) female results, (right) male results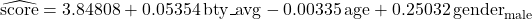
$\widehat{\text{score}}=3.84808+0.05354\,\text{bty\_avg}-0.00335\,\text{age}+0.25032\,\text{gender}_\text{male}$ .

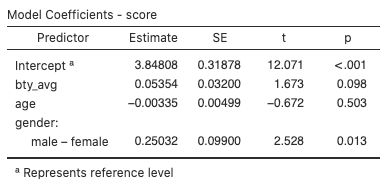
Figure 2: Model coefficients for professor evaluation
Adjusted R² is 0.0597.

Figure 3: Model fit measures for adjusted R²
One predictor, gender (p-value = 0.013), has a significant coefficient at the 0.05 significance level and two predictors, bty_avg (p-value = 0.098) and age (p-value = 0.503), do not.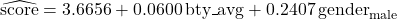
$\widehat{\text{score}}=3.6656+0.0600\,\text{bty\_avg}+0.2407\,\text{gender}_\text{male}$ .

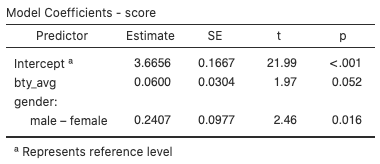
Figure 4: Model coefficients for professor evaluation model
Adjusted R² has increased to 0.0654.

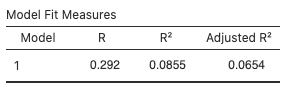
Figure 5: Model fit measures for adjusted R²
One predictor, gender (p-value = 0.016), has a significant coefficient at the 0.05 significance level and one predictor, bty_avg (p-value = 0.052), does not. However, the coefficient for bty_age is very close to 0.05.
For males, 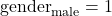$\text{gender}_\text{male}=1$ , so the estimated regression equation is $\widehat{\text{score}}=3.6656+0.0600\,\text{bty\_avg}+0.2407(1)=3.9063+0.0600\,\text{bty\_avg}$ .
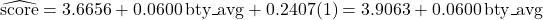We expect score to increase by 0.0600 for each additional one-unit in bty_avg, holding gender fixed. This interpretation is the same for males and females.
Given that the coefficient for bty_avg is slightly above 0.05, the model accounts for only 8.55% of the variation in score, and the average increase in score for a one-unit increase in bty_avg is only 0.0600, the association between score and bty_avg in this model is not really of much practical significance. Other factors not considered here are likely to be of much greater relevance.